How to Teach Fractions the Easy Way
Teaching and working with fractions can be extremely challenging. So much of it is non-tangible, like imagining what ⅚ of a large group looks like. But there are ways to make these more theoretical skills accessible through food, toys, and easy to create activities!
Cook Up a Storm
Everything about cooking and baking is related to fractions! Just go read a recipe book. It is littered with fractions of a cup, tablespoon, and other measuring tools.
For younger learners, just practicing talking about fractions can be useful. While you are cooking, practice pouring out the item piece by piece. If you need ¾ cup flour, pour ¼ at a time. Explain: there is one whole cup of flour and it has been divided into four equal parts. I know it is four equal parts because of the denominator (bottom number). We need just three of those parts. If we had all four of the four parts, we would have a whole cup!
Older students can practice creating similar fractions or doubling and tripling recipes.
To convert a fraction into a similar fraction, you need to understand or be able to work with multiples. For example, ½ tbs of salt is needed to make chocolate chip cookies. Next, find a multiple of 2 (the denominator). I picked 4 (2×2=4). Now do the same thing to the numerator that you just did to the denominator. Since I multiplied 2 by 2, I need to multiply 1 by 2. ½ became 2/4! Since you increased both parts of the fraction equally, it didn’t become larger in quantity, just in pieces of the whole!
To double or triple a recipe, the math is almost exactly the same as creating a similar fraction. However, instead of working with both the numerator and the denominator, this time you will only multiply the numerator. For example: I am doubling a batch of cookies. The original recipe calls for ¾ cup of chocolate chips. I know that multiplying by 2 will double the answer. I multiply the numerator by 2 (3×2=6), so I need 6/4 cup of chocolate chips. With this, you can also practice converting to mixed numbers.
To convert from an improper fraction to a mixed number, divide the numerator by the denominator. 6 ÷ 4=1 remainder 2. You have one group of four parts which makes a whole, and two out of the four parts needed to make another whole OR 1 2/4. You could practice creating similar fractions and reduce 2/4 to ½, too.
Creating Fractions
This is probably the easiest, and most fun, thing to do. Get literally anything that can be divided into equal groups: pizza, toys, leaves, seriously anything. Then, divide it!
For toys, you can practice making groups of equal size/amount and creating fractions of a group. Once you have created your groups, explain: We had 30 toy cars and put them into six equal groups. One of these groups equals ⅙ of the total group of cars. There are five cars in each group. That means that ⅙ of 30 equals 5!
If you use food (pizza, cake, anything that can be slices), divide it among your family members. Let’s say that you have 4 people in your family, you would divide the pizza into fourths, eighths, twelfths, sixteenths, or any other multiple of four. Then, have each person count how many total pieces he or she ate. Finally, chart the total amount. Dad ate 6 of the 16 pieces, so he ate 6/16 of the pizza. This is also a great opportunity to practice reducing fractions. If Tommy ate 4 of the 16 pieces of pizza, how much pizza did he eat in simplest terms? 4 and 16 can both be divided by 4, which turns 4/16 into ¼.
Adding and Subtracting Fun
Adding and subtracting fractions can be challenging. However, you can use food and Legos to help you out.
An easy way to add with fractions is to use oil and water. If the denominators of the fraction are the same, you can just measure out the fractions. Make one fraction water and the other fraction oil. Pour them, one at a time, into a separate measuring container. I like to use beakers or tall liquid measuring cups for the final measurement. After you have poured your two liquids into the final cup, just check how much total liquid there is.
You can also practice working with volume measurements with this same method. With a variety of containers in cups, pints, quarts, and gallons, you can pour water from one container into another. This is a fun way to make the conversions between measures more real for children.
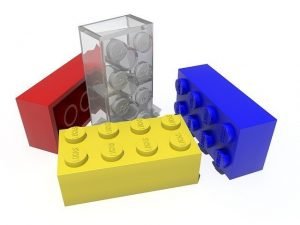
Legos are fun to play with and are easy to find. As a bonus, they are already conveniently divided into fractions. All you need to do to make it even more obvious is to add some lines with permanent marker. Using your marked up Legos, you can add, subtract, and compare fractions. Highlight ⅗ of one block and ⅖ of another block, then ask your student to count up the highlighted fifths (7/5). From here you can convert to a mixed number by dividing the numerator by the denominator. With these same two blocks, you can compare ⅖ and ⅗ visually. You can also subtract: How many more fifths is ⅗ than ⅖? Your student should be able to count up the fifths and see that ⅗ is ⅕ more than ⅖. Best of all, there are Lego blocks of various sizes and you could even combine smaller blocks to equal a “whole” larger block.
Word Problems and Other Skills
Word problems involving fractions are challenging, and not just for kiddos. Fractions require you to read carefully, review all the known information, determine what is being asked, and then pick one or more strategies to reach the solution(s). However, you can use simple “talk it out” strategies to work through these problems together.
Below, I’ve written two different kinds of word problems students might encounter involving fractions AND the talk it out solutions that I would use in class to model my thinking process for students. While these are only two samples of an infinite variety of problems, kids will come across these type frequently.
Example 1: I made 24 cupcakes for my Girl Scout meeting. ¼ of the cupcakes are chocolate, the rest are vanilla. How many cupcakes of each flavor are there?
Solution 1: I know to divide by 4 because 4 is in the denominator of my faction. 24 ÷4=6. So one group, ¼, of the cupcakes would be 6. 6 cupcakes are chocolate. I know that each fourth will have 6 cupcakes in it, and if I have already counted ¼, I have ¾ left. 6×3=18 vanilla cupcakes.
Example 2: Susan has 45 stickers in her sticker pad. Yesterday, she shared ⅕ to Jesse and she stuck 1/9 of the original 45 onto a picture. How many stickers does she have left?
Solution 2: This requires two different steps. First I need to figure out ⅕ of 45. I know to divide by 5 because of the denominator. 45 ÷5=9. Susan gave 9 stickers to Jesse. I also need to figure out 1/9 of 45. 45÷9=5. Susan stuck 5 stickers onto her picture. 5+9=14. 45-14=31 stickers remaining.
Fractions are everywhere, but we do need to be creative and resourceful to make the connections between everyday activities and our student’s curriculums!
Meg Flanagan is a special and elementary education teacher who holds an M.Ed in special education and a BS in elementary education. In addition to classroom experience, she has also worked in private tutoring and home schools. Meg is passionate about education advocacy for all children, but especially for children with special needs and children of military and state department personnel. You can find Meg online at MilKids Education Consulting, as well as on Facebook and Twitter.
